Formula
Assuming we know the derivative of tan(x) is sec 2 (x): Let y = arctan(x) so that x = tan(y). Differentiate both sides with respect to x to get: 1 = sec 2 (y) dy/dx. Now use the identity. Sin 2 (y) + cos 2 (y) = 1. Divide by cos 2 (y) to get. Tan 2 (y) + 1 = sec 2 (y) Use the substitution tan(y) = x to get. Sec 2 (y) = 1 + x 2. Calculate the derivative of the function (y = arccos x arctan x ) at (x = 0. ) Example 9 Using the chain rule, derive the formula for the derivative of the inverse sine function.

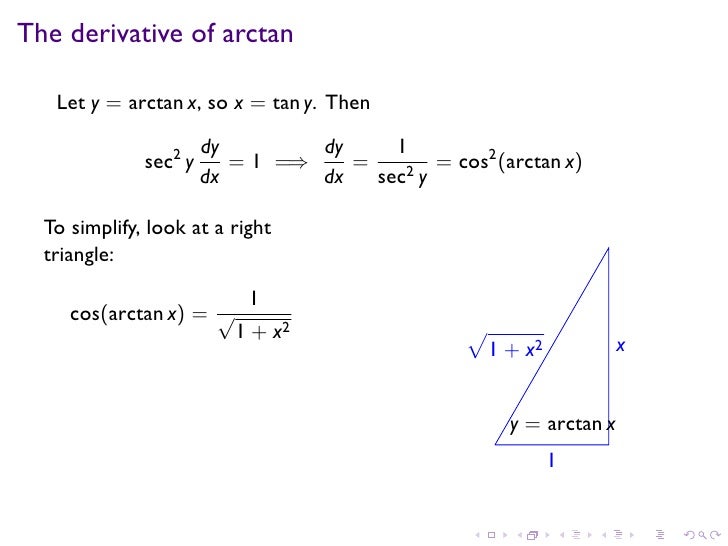
The inverse tangent — known as arctangent or shorthand as arctan, is usually notated as tan-1 (some function). To differentiate it quickly, we have two options: 1.) Use the simple derivative rule. 2.) Derive the derivative rule, and then apply the rule. In this lesson, we show the derivative rule for tan-1 (u) and tan-1 (x). Differentiating Arctan(x) It's great fun to differentiate Arctan(x)! Here are the first 20 derivatives. (Notice that where n represents the number of the derivatives and t represents the number of terms in the expression, as n-infinity, t-infinity.).
$dfrac{d}{dx}{, tan^{-1}{x}} ,=, dfrac{1}{1+x^2}$
Introduction
The inverse tangent function is written as $tan^{-1}{x}$ or $arctan{(x)}$ in inverse trigonometry, where $x$ represents a real number. The derivative of the tan inverse function is written in mathematical form in differential calculus as follows.
$(1) ,$ $dfrac{d}{dx}{, Big(tan^{-1}{(x)}Big)}$
$(2) ,$ $dfrac{d}{dx}{, Big(arctan{(x)}Big)}$
The differentiation of the inverse tan function with respect to $x$ is equal to the reciprocal of the sum of one and $x$ squared.
$implies$ $dfrac{d}{dx}{, Big(tan^{-1}{(x)}Big)}$ $,=,$ $dfrac{1}{1+x^2}$
Alternative forms
The differentiation of the tan inverse function can be written in terms of any variable. Here are some of the examples to learn how to express the formula for the derivative of inverse tangent function in calculus.
$(1) ,$ $dfrac{d}{dy}{, Big(tan^{-1}{(y)}Big)}$ $,=,$ $dfrac{1}{1+y^2}$
Derivative Of Arctan(y/x)
$(2) ,$ $dfrac{d}{dl}{, Big(tan^{-1}{(l)}Big)}$ $,=,$ $dfrac{1}{1+l^2}$
Derivative Of Arctan U
$(3) ,$ $dfrac{d}{dz}{, Big(tan^{-1}{(z)}Big)}$ $,=,$ $dfrac{1}{1+z^2}$
Derivative Of Arctan U
Proof
Derivative Calculator
Learn how to derive the differentiation of the inverse tangent function from first principle.